The Fierce Urgency Of Now

The Fierce Urgency Of Now:

“You have to let ideas win, not hierarchy.” – Steve Jobs.

          Mark Suppes is out.  The community is devastated to see him go.  Why did this happen?  Money - Mark needed support, and he never got it.  This has become very common; even for the best researchers.  Dr. Klein had an Ivy League PhD, publications and experience from Oxford [47].  Any idea from him had been scrutinized.  Why then, did it take him ten long years to get funding? 

          Money is always a tough issue.  The US spends about 400 million on fusion a year.  Recently, this money has been changing directions.  NIF is declining.  Its budget is down 20% for next year.  This is probably because the machine failed to ignite.  Next, ITER is rising.  It will eat over half the budget.  Meanwhile, domestic programs are ending.  MIT’s tokamak may fall in October.  We are sorry to see them go – but we gave them 41 years, and there are still no tokamak power stations [40, 41]. 

          When a 14 year old kid can fuse atoms in his garage – it screams revolution.  It says that fusion energy will have nothing to do with a big machine in France.  The final solution may not be a polywell.  But the answer lies somewhere down that road.  To get there, we need broader funding.  Why focus on two well-worn ideas, when alternatives exist?  The ITER machine got a 75 million increase this year.  That could have funded ten new ideas.  If we stay on this path; we will never get there.

          We need to get there.  Burning carbon is killing our world.  It causes global warming.  It is unsustainable.  We know we need to change.  Sustainable energy is only way out.  To win, it must scale.  To win, it must be cheaper than carbon.  Fusion energy could be that solution.  Today, we have brand new ways to get there.  So, what are we waiting for?

"Vision is the art of seeing what is invisible to others." –Johnathan Swift

Executive Summary:

          This post reviews work measuring electrons inside a polywell.  The Australian team used a new biased probe to find electron density, temperature, speed and the local voltage.  This data came from reading the current drawn by the probe.  Due to cost limitations, this analysis could not be automated.  Precise equations for the plasma and probe were fitted to the data, ad hoc.  A new 4” aluminum polywell was used.  Results from simulations are also presented.  Five tests were done.  First, a beam was measured, and this bench marked the probe and analysis.  Second, the center was checked with and without a magnetic field.  The results supported electron trapping.  Next, measurements were made along the x-axis and this also showed trapping.  The fourth test looked at the effect of the ring field on the electron cloud.  Data showed that the potential well rises with ring strength.  Finally, the impact of the electric field on the potential well, was tested.  As more and faster electrons are injected, the center gets more negative.  This ends when speedy electrons, start to escape the ring field.  The details of the paper are in the appendix, and select data can be downloaded here.

News:

          After an ode to the fusioneer was posted in April, work focused on the polywell 101.  The film explains the machine using a voiceover and a whiteboard.  It addresses: mechanism, design, operation and challenges.  That work was part of a collaboration, with the folks at Talk-Polywell.  Thanks to everyone who helped out.  In May, the world passed 400 ppm of carbon dioxide - a clear sign that we need new energy sources.  Also in May, Dr. Joe Khachan published another paper in physics of plasma.  This post will review that work.  That month, the Turkish atomic authority published a fusor paper.  Their machine was about a foot across and produced 2E4 neutrons per second [44].  This was done with an 85 kilovolts drop across the cages.  This implies they used some costly equipment.  Those specs put the Turkish team near the high end of the amateur scale.  They have better equipment; but Richard Hull has gotten more neutrons.  Photos of their equipment are shown below. 

The Turkish paper mentions polywells and penning traps as the next step [44].  We cannot know if they will build one (their vacuum chamber is not appropriate) however, it is a possibility.  Bottom line: we now have a new group in the game.  In August, the Polywell was included in an MIT competition for solutions to the climate crisis.  You can vote for it, by logging in here.    

“I hoped that a venture capitalist would get excited and say: Let’s Do This!  It never happened.” – Mark Suppes

Review of May 2013 Paper:

Overview:

          This goal of the Khachan work is to prove that electrons are trapped in a Polywell.  This has been shown before [17] but we can now get more details about the electrons.  This paper will measure the electrons density, temperature and speed inside a polywell.  It will also connect this to the magnetic and electric fields in the machine.  This work is exciting, but the results are limited.  The team did not have tons of cash to work with - and it shows.  Their polywell was small and it had a low power source.  Their probes and analysis were homemade.  They squeezed as much data out of this setup as they could – but they will need more funding to go farther.  We need to give them that support.

          These new details are because of a Langmuir probe.  For ninety years, these probes have been used to measure plasmas [33].  The team is using a brand new biased probe.  It is a thin tungsten wire with a voltage applied to it.  This lets them collect new data about the electrons.  This data will be used to build the case for electron trapping.  The team will argue this case - as if they were lawyers in a courtroom.

          Their argument will be structured around four experiments.  The first is a test case.  The probe is stuck in the center of a non-working polywell.  It will measure a beam of electrons as they pass by.  This test will bench mark the probe and analysis.  The second test compares a working and non-working polywell.  Predictably, when a magnetic field is added, it changes everything.  The third test moves the probe around during operations.  The electrons are measured along the x-axis. This will show the environment at different places inside the cloud.  The fourth test has two parts.  First the voltage drop in the center is monitored while the ring strength changes.  Second, the voltage drop is measured while the electric field changes.

 

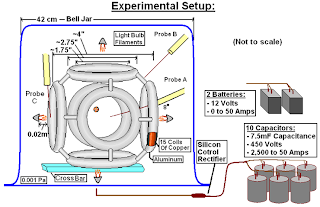
Experimental Setup:

           These new measurements require a new setup.  About half the setup changed from their 2010 paper.  They used the same vacuum chamber and polywell mount [27, 16, 17].  They powered the rings with the same set of capacitors; although two car batteries were added on.  What are new - are the probes, injectors and polywell.  Three probes were added.  Each probe was a long, thin wire, with backup equipment.  Details on the probe are included below.  The team used six light bulb filaments for injectors.  When these wires heat up, electrons are kicked off.  They fly towards the positive polywell and get trapped.  These injectors make plasma, of a known density.  This was vital for data analysis.  Lastly, they built a new aluminum polywell.  This setup is pictured below. 

A New Polywell:

          Like their old Teflon polywell - this aluminum device is also about the size of a coffee cup.  We used photos to estimate the size [23].  The rings were 2.25 inches in diameter.  Each ring had a cross section, a half inch in diameter.  The rings were smooth aluminum shells, with 15 coils of coated copper wire inside.  The smooth surface is critical for success.  The shells were made by metal spinning [17].  A thin disc of soft aluminum is centered on a lathe.  The disc is spun at high speeds.  A wedge is pressed into the metal.  As it spins, the metal curves around the wedge [22].  This is an inexpensive way to make the device.  A photo is shown below.

Spinning made twenty-four parts that needed to be assembled to make the Polywell.  In the picture, this is done with metal ties and soldering.  During experiments, ties or clamps were used to hold the rings together [27].  This was needed because during peak use, each ring was pushed apart with 15 pounds of magnetomotive force [appendix].  Unfortunately, the clamps pierce the stream of recirculating electrons.  This creates problems [29].  Electrons that touch the clamps could be lost.  Losses are made worse, because the clamps and the rings were all held at a positive voltage.

Improvements:

          How could we improve this device?  First, the clamps cannot remain.  With no clamps, one must bond the rings together.  Unfortunately, spot and arch welding are both out.  They would melt everything and make a mess of delicate parts.  Soldering is preferable.  This narrows the options for ring materials.  One possibility is 3003 aluminum.  This is pliable for spinning and good for soldering [24, 19].  The metal can be soldered at 220C [25].  This temperature will not melt the copper wire inside, but it may burn the wire cladding.  To do this, the shells would be heated on a hot plate and a solder wire would be applied [26].  Afterwards the part may be sanded for a smooth surface. The final device would be made of aluminum, with traces of tin and silver.  Materials selectors will also need to consider: neutron activation, thermal conductivity and magnetic transparency. 

Langmuir Probes:

Background:

          These probes were made by Nobel laureate Irving Langmuir.  You stick a thin wire into the cloud.  As the positive and negatives touched the metal, a current is drawn from the wire.  This is a cylindrical probe [36].  You biased this wire at some voltage.  As the wire changes from negative to positive, the current also changes.  This signal is known as a current-voltage or IV curve.  From this data: the plasma density, energy, charge and potential can be found [37].  But this is not easy.  You need to do some mathematic acrobatics to get it to work [34].  Reading this signal is actually its own field of study.  Normally, you outsource this work to a company with software and fancy tools [35].  But the team had no money.  They had to tackle this analysis by hand.  Each wire-plasma interaction was analyzed ad hoc.  Here is an illustration of this process.

There are some key facts about this analysis.  First, voltage changes throughout a plasma cloud.  This is called the local voltage.  The wire must be stuck in to measure this voltage.  The volume around this wire is called a sheath.  This is a plasma filled region.  The plasma in the sheath behaves differently than the rest of the cloud.  This behavior comes in two types.  In type one the wire is positive compared to the cloud.  This means that the wire voltage is greater than the local voltage. Here, electrons cluster around the wire.  They are attracted to the positive charge.  The sheath is filled with electrons - and all of them touch the wire [42].  In type two, the wire is negative to the cloud.  Here, electrons are repulsed.  Any negative charge that touches the wire, must have overcome this repulsive field.  To do this, they need a minimum velocity.  This velocity can be estimated and must be included in the analysis.  These two types are illustrated below.

Rules for Langmuir Probes:

          For each test, the team had to read this probe data.  This meant changing IV curves into electron information.  A specific analysis must be done for each case - but there are some general rules.  First, the sheath must be symmetric around the wire.  This ends up limiting the analysis done in this paper.   Second, the wire must appear as infinite cylinder, with no width, to the electron.  This is needed for the math but it forces the probes to be long thin wires.  Third, the electrons in the sheath must proceed directly to the wire.  If they collide beforehand, it messes up the analysis.  This causes the team to only work with low density plasmas.  If they had the cash, high density probing would be possible [48].  It also places rules on the size of the probe, the polywell and the plasma Debye length. These rules are shown below [34, 16].  These rules are designed so that the current to the wire depends on two values: the initial velocity and number of electrons around. 

Math and Data:

         Nobel Prize winner Irving Langmuir worked all these rules out [42].  He reasoned that if electrons follow his rules, he could predict the current to the probe.  His analysis relied on the plasma velocity and the type of probe.  Using these conditions, he designed math to go with them.  That is powerful.  It means you can fit equations to data.  In doing so - you can find the local voltage, density and energies.  This is what Khachan will do.  They will stick wire probes into four different polywell setups.  The probes will draw current.  This data will then be fitted to specialized equations.  Based on the fit, plasma information will be found.  Fitting requires two expressions.  The first is for the wire and the second is for the plasma.  The wire equation is a general cylindrical probe equation that applies to all wire probes [42].  This expression is shown below.  

Plasma Equations:

          The equation for the plasma is a velocity distribution for the electrons.  Working out the velocity distribution is the hardest part of this paper.  It must be done on a case by case basis.  The team has three velocity distributions to choose from.  The first is for normal electrons with a bell curve of velocities.  They never use this - and that is a key fact.  None of the electrons measured here appear to have bell curves of energy.  The second is for a beam of electrons, all near the same speed.  This “beam analysis” is used several times.  The third expression is for electrons in a cloud, all near the same speed.  The challenge is picking the right expression for the plasma tested.  The velocity and probe equations are combined and integrated.  This leads to nasty integrals which can only be solved numerically.  This work is shown in the appendix.  Their solution predicts current to the probe at a given voltage.  When equation and data are fitted together, measurements pop out.

Test I: A Beam

          The team started with a simple experiment to verify their analysis works.  They measured the electrons in a beam.  We expect beam electrons to be monoenergetic [45].  The electrons should all be at the same temperature and the thermalization ratio should be low.  This is a situation where we know what we should get.  If the data matches prediction, it benchmarks the probes and the analysis.

          The setup they had was simple.  They put one emitter seven centimeters away from polywell center.  When electrons were kicked off, they become attracted to the rings.  This is because the rings are at a positive 150 volts.  A probe is stuck in the center of the Polywell.  This wire will absorb some of the electrons, making an IV curve.  The team expects a certain IV curve.  An illustration of this is shown below.

Basic models can predict much about this setup.  First, the electrons are moving along the x-axis.  Along this axis, the polywell is symmetric.  Therefore we can ignore four of the rings.  The two remaining rings are treated like flat discs.  These discs are loaded with positive charge.  This charge makes the rings positive and attracts the beam.  The field made by one ring can be predicted with a simple equation [38, 39].  The total electric field is a superposition of two disc and this can predict electron speed.  The model is explained in detail in the appendix.  Our model predicts about one third the measured electron speed near the probe.  That is pretty good.

Beam Velocity Distribution:

          Electrons in a beam can be modeled as having the same energy [43].  But, real life is different.  The energy will spread out some.  Langmuir developed the original equation [42] but the team had to adjust this for their work.  Because the probe was at an off angle – theta – to the beam, the expression was changed slightly.  The resulting velocity distribution is shown below.  This equation was combined with the probe equation.  The final expression was a double integral and is shown in the appendix.  This expression should predict the current to the probe.

The team solved this double integral numerically.  They then fitted it to real data.  We tried to duplicate this, but failed due to lack of time.  The plan is to upload this MATLAB code so the community can try it.  When the model matched the data, the plasma properties were found. 

          This yielded lots of information.  Generally, the beam was monoenergetic with a spreading bell curve around one speed.  This is important.  The electrons were not thermalized.  If they had been, the probe data would looked different.  The beam had a velocity of 6.05E6 meters per second.  Our model predicted a speed of 1.7E6 – which is the same order of magnitude.  The electrons had a density of 1E14 electron per square meter.  This matched typical plasma made by heated filaments.  In addition, the voltage around the probe was 125 volts.  This is sensible, since the nearby rings were at 150 volts.  This local voltage becomes a key measurement the team will use later on.  Finally, the electrons were at a temperature of 9,279 degrees.  They had been heated by the electric field around the rings.  WB6 heats ions to fusion conditions, using a similar mechanism.  Unfortunately, we cannot extend Khachans results to WB6, since the machines are so different.

Test II: Polywell Off and On:

          The second test compares a working and non-working Polywell.  A probe is put in the center.  Electrons fly in. They are attracted to the positive rings.  The rings are held at 112 volts.  In first test the polywell is off.  Here one beam of electrons passes by the probe.  The data is analyzed in the same way as test one.  Next, the device is on.  Now a magnetic field is added and this changes everything.  Electrons are moving through a null point.  We can expect some behavior.  They should move in straight lines [46].  This will scatter them and that leads to their eventual loss. Electron leakage is a big problem.  They are likely uniformly spaced and at similar energies [16]. 

Cloud Velocity Distribution:

          These conditions lead to a electron velocity expression.  This equation is brand new.  It appears to be composed solely for this paper.  Hence, they must prove that it is acceptable.  First they use simulations.  The electron speed is measured inside a two dimensional polywell simulation.  Results show that electron speeds are lower in the center.  This supports the idea that electrons are cold in the center.  The simulation is described in the appendix.  Simulated speeds are a good fit for this expression.  Following that, the team uses two other math checks in support.  First, when there is no average speed, the expression becomes the bell curve.  Second, when there are no thermalized electrons, it predicts every electron at one speed.  The math checks out.  The equation is shown below.  It is inside the x, y and z coordinate system.  To combine it with the probe one, it seems you need to change coordinate systems.  

Options:

         With this equation, khachan has made three IV curve models that he can use.  The first is for electrons with a bell curve of velocities.  He never tells us what this is.  But none of the data fits this.  That is important.  The second is for electrons in a beam.  This fit is used in test one.  When the polywell is off, it is the only model which fits the data.  This is sensible.  The last model is the brand new cloud equation.  This is for a tight curve, centered on one speed.  When the polywell is turned on, this should be the only model to fit the data,.  Unfortunately, this is not the case.  Both the beam and cloud models fit the data.  This means that he cannot be 100% sure about the results.  The paper acknowledges this flaw [16].  But, he uses simulation and theory [46] to support for the “cloud analysis” when the polywell is on.  Results from these fits are shown below.  The working polywell results include the beam and cloud analysis.

           Test two tried to prove that a cloud of electrons has formed.  Unfortunately, the data is inconclusive.  At first it looks promising.  The voltage has dropped.  This means that when the polywell is on, the center gets more negative.  This implies a cloud of electrons is trapped there, which lowers the voltage.  However, the density remains the same.  This is hurts the idea – if a cloud of electrons is concentrated, the density should rise.  Lastly, the temperature and average electron speed remains unchanged between tests. 

Test III: Moving The Probe:

          Test three involves moving the probe outward.  The polywell is fully operational - with both a magnetic and electric field.  The probe moves through the center - and data is taken at different points.  The goal again is to find a cloud of electrons.  The evidence for this is a dip in voltage.  As the probe travels, it gets into denser magnetic fields.  This changes the electron behavior.  In the center, the electrons move in straight lines - they have an infinite gyroradius.  Outward from the center, the electrons start corkscrewing.  As the field increases, they spiral in tighter orbits.  The tightest orbits are at closest to the rings.  The team can estimate the orbital radius; this is described in the paper.  The electron behavior is illustrated below.

Experiment:

          This test must have been a pain.  If the probe was inside the bell jar, then each data point would require a full machine run.  The polywell was held at 109 positive volts to attract these electrons.  Each ring had 7950 ampturns in it.  The probe would then be moved, and the whole test repeated.  All that could have taken weeks.  The probe moved along the ring axis.  It went from zero to three and a half centimeters outwards. This is about seventy percent the distance to the rings.  They made 13 measurements - and these are indicated by the red dots above. 

Analysis:

          The team used the cloud velocity distribution.  This is the same velocity distribution used in test II.  This should work in the center – but it will become a crummier fit as the probe moves outward.  The reason for this is the magnetic field.  The field changes the electron motion.  This, in turn, forces the plasma around the wire to be non-uniform.  The sheath becomes asymmetric and skewed.  This breaks Langmuir’s rules.  Hence, the analysis starts to be a bad fit for the data.  Through estimating the radius of gyration, the group reasons that measurements are good to 2 cm out from the center.  After this, the uncertainty grows.  This two centimeter rule becomes important in test four.  Measurements were taken out to 3.5 cm.  The local voltage is plotted below, with error bars. 

          We have a couple of problems with this data.  First, what is missing is telling.  For each measurement, they must have known the density, temperature and average velocity – but that data is not included.  They did give us numbers from an extra test that was not included in this set.  Those values are sensible: at 1.8 centimeters they measured electrons at 10,439 degrees kelvin with a density of 1.3E14 particles per cubic meter and a mean speed of 2.8E6 meters per second.  These figures are fine and are shown in the appendix.  But, those measurements were not connected with the data plotted above.  Finally, a magnetic field is listed at each measurement location.  But, this was likely estimated.  

Results:

          Test III proves that electrons were trapped in the center.  It also shows where they are.  A drop of about 80 volts was measured.  This spanned from the positive rings to device center.  This value is sensible; such a sharp drop is consistent with results from test II.  The drop tells us there is a cloud of negative charge.  Inside this cloud there is a 6.6 voltage dip in the middle.  That dip, points to a dense core of electrons in the dead center.  This structure is illustrated below.  We must take this with a dose of skepticism.  The analysis has its caveats and the polywell they used is limited in size.  Also, we must assume that this is radially symmetric.  However, these results show a cloud of negative charge trapped.  This conclusion is reinforced by test IV.

Test IV: Finding Correlations

Part A: Trapping and B-Field:

          Test four links the electron cloud to the magnetic and electric fields.  It has two parts.  In part a, we watch the cloud as the magnetic field varies.  In part b, we vary the electric field.  These tests require two probes to be used at the same time.  One sits at the center.  The other is as far away from the center as possible.  This is 1.8 centimeters away.  Beyond this distance the probe analysis begins to fall apart.  Both probes monitor the local voltage.  If the center is negative to the outside probe - a cloud has formed.  

          All probe data will be fitted using the cloud velocity distribution.  The polywell is held 116 volts positive to the emitters.  All six emitters are used.  They emit 2.6 milliamps of electrons.  The rings were likely powered by car batteries.  Twenty six readings were taken as more current flowed into the rings.  As the number of ampturns rose, the magnetic field increased.  The axis field peaked at 26 milliteslas.  The data is shown below.  As the power increases - a pattern emerges.  The pattern is that the center is more negative than its surroundings.  This is strong evidence that the polywell is trapping electrons. 

Part B: Trapping and E-Field

          Now, the effect of the electric field on trapping is measured.  The magnetic field is held constant and it is very high.  The rings are at about seven times the strength as part a.  The axis field is at 0.16 Tesla.  This allows many electrons to be trapped inside the polywell.  The same two probes are used inside the machine.  The team now changes the voltage the polywell is held at.  They vary the rings from 93 to 122 positive volts.  In doing this they are raising the surrounding electric field.  This has an interesting effect on the cloud.  The results are shown below.

Part B Results:

          The data has two sections.  In section one, raising the electric field means more trapping.  This makes sense.  A deeper field means more electrons fly into the center.  This leads to bigger clouds.  The data shows this.  The center gets negative compared to the outside.  This changes in section two.  As the electric field rises the electrons speed up.  This makes sense.  If the particle is accelerated with a stronger field it should move faster.  Eventually, the electron moves so fast it cannot be held in by the rings.  The electrons escape.  They have so much speed they can fly out of the ring field.  The cloud is leaking electrons - like a cup which is overflowing.  The data does reflects this.         

          A couple of comments.  First, the team should have also reported the electron speed, since they had the data.  Those numbers would help their speed argument.  Why did they exclude these numbers?  Second, there is another way to adjust electron speed.  Moving the emitter farther away will also raise electron speed.  We showed this in a previous post.  Lastly, this analysis is preliminary.  Since this technology is so new, many more tests are needed.  We will probably discover many more relationships.  But, we need to do those tests.

Conclusion:

         

          This paper hints at the kind of work we need in the future.  We need to connect input and output variables.  This paper starts to do that.  By doing electrons only, they have simplified the problem.  Here are some important electron only, input variables:

1. The electric field around the Polywell [volts].

2. The Magnetic field made by the rings [ampturns].

3. The distance from the probe to the rings [meters].

4. The amount of electrons injected [amps].

5. The shape, design and number of rings. 

The output is the potential well.  We want to maximize this.  We also want to hold this overtime.  We need to tune the above five inputs to do that.  This paper shows some basic relationships.  As the electric field increases, so does the well.  As the magnetic field increases, so does the well.  We also know that as the emitters move away, the electron speed rises.  The team needs more funding to try larger machines - they have a 13" polywell running now.  In addition simulations can be used to try lots of designs we do not need to build.  This is exciting.  It is very likely that the polywell has a operational sweet spot.  If we can find this, we may find fusion energy.  If can do that, we can change the world.   

References:

1.               Tibbets, Dan. "Debate on Electron and Ion Temperature inside the Polywell." Talk-Polywell.org. Talk-Polywell.org, 28 May 2013. Web. 19 June 2013. .

2.               Krall, Nicholas A., M. Coleman, and K. Maffei. "Forming and Maintaining a Potential Well in a Quasi Spherical Magnetic Trap." Physics of Plasma. American Physical Society, 6 Oct. 1995. Web. .

3.               "Energy/Matter Conversion Corporation in Santa Fe, New Mexico Headquarters Location." Www.findthecompany.com. Find the Company Inc, 18 June 2013. Web. 19 June 2013. .

4.               Bussard, Robert. Method and Apparatus for Controlling Charged Particles. US Patent Office, assignee. Patent EP0242398. 29 Oct. 1985. Print.

5.               Tidman, Derek A., and Nicholas A. Krall. Shock Waves in Collisionless Plasmas. New York: Wiley-Interscience, 1971. Print.

6.               "Nicholas Krall." Pipl Directory. Pipl Inc., 19 June 2013. Web. 19 June 2013. .

7.               Krall, Nicholas A. "MONITORING ENERGETIC A-PARTICLES IN A FUSION DEVICE." SBIRSource.com. SBIRSource.com, n.d. Web. 19 June 2013. .

8.               Corbett, Bob. "Fusion Power Nearer." Copley News Service, Reading Eagle [Berks County, PA] 30 Nov. 1980: 44. Print. http://news.google.com/newspapers?nid=1955&dat=19801130&id=JeUiAAAAIBAJ&sjid=mKIFAAAAIBAJ&pg=6368,6517585

9.               "KRALL ASSOCIATES." Bizapedia.com. Bizapedia Inc, 2012. Web. 19 June 2013. .

10.           “The Polywell: A Spherically Convergent Ion Focus Concept” N. Krall Fusion Technology, Vol 22, August 1992.

11.           Meeting Complaint over the fusion energy foundation, 12/16/1980

12.           Should Google Go Nuclear?" R.W. Bussard. Google Videos. 9 Nov. 2006. 20 Feb. 2009. 32:33.

13.           Watrous, J. J. "Publications List." FTI Publications. University of Wisconsin-Madison, n.d. Web. 19 June 2013. .

14.           Duncan, Mark. "Should Google Go Nuclear?" Askmar. Www.askmar.com, 24 Dec. 2008. Web. 3 July 2012. .

15.           Xiong, Helen. "Presolicitation Notice Plasma Whiffleball 8.0." NECO Synopsis Database. The US Navy - NAVAL AIR WARFARE CENTER, 10 Mar. 2012. Web. 19 Apr. 2012. .

16.           Carr, Matt, and Joe Khachan. "A Biased Probe Analysis of Potential Well Formation in an Electron Only, Low Beta Polywell Magnetic Field." Physics of Plasmas 20.5 (2013): n. page. Print.

17.           Carr, Matthew, and Joe Khachan. "The Dependence of the Virtual Cathode in a Polywell™ on the Coil Current and Background Gas Pressure." Physics of Plasmas 17.5 (2010). American Institute of Physics, 24 May 2010. Web.

18.           "3003 Aluminum Material Property Data Sheet - Product Availability and Request a Quote." 3003 Aluminum Material Property Data Sheet - Product Availability and Request a Quote. Metal Suppliers Online, LLC, 1995 - 2013. Web. 19 June 2013. .

19.           "Aluminum Alloy 3003." McMaster-Carr. McMaster-Carr, 18 June 2013. Web. 19 June 2013. .http://www.mcmaster.com/#aluminum-alloy-3003/=n8w1bu

20.           Rider, Todd H. "A General Critique of Inertial-electrostatic Confinement Fusion Systems.  "Physics of Plasmas (1995): 1853-872. Web.

21.           "Metal Spinning." Wikipedia. Wikimedia Foundation, 31 May 2013. Web. 19 June 2013. .

22.           Khachan, Joe. "OVERVIEW OF IEC AT THE UNIVERSITY OF." 14th US-Japan Workshop on IEC Fusion. N.p., 19 Oct. 2012. Web. http://www.aero.umd.edu/sedwick/presentations/S1P3_Joe_Khachan_Presentation.pdf.

23. "Aluminum Alloy Properties." McMaster-Carr. McMaster-Carr Inc., n.d. Web. 19 June 2013. .

24.           "Soldering Types to Purchase." McMaster-Carr. McMaster-Carr Inc., n.d. Web. 19 June 2013. <http://www.mcmaster.com/#general-purpose-solder/=n8ycrf>.

25.           "Send To A Friend Aluminum Solder Wire 96 Sn/4 Ag .062 Flux Core." Aluminum Solder Wire 96 Sn/4 Ag .062 Flux Core Aluminum Solder Wire Solder Wire Main Section SRA Soldering Products. SRA Soldering Products, 24 Jan. 2013. Web. 19 June 2013. .

26.           Tibbets, Dan. "View Topic - What Is the Best Material for the Rings?" Talk-Polywell.org. Talk Polywell, 2 Nov. 2011. Web. 2 Nov. 2011. http://www.talk-polywell.org/bb/viewtopic.php?t=3373.

27.           Joe Khachan, Private Communication June 19, 2013.

28.           "Ornamental and Decorative." UK Metal Spinning. Steel Spinng Ltd, 22 June 2013. Web. 24 June 2013. .

29.           Rider, Todd H. Fundamental Limitations on Plasma Fusion Systems Not in Thermodynamic Equilibrium. Thesis. Massachusetts Institute of Technology, 1995. Print.

30.           Suppes, Mark. "Deepest Potential Well Yet: 43 Volts." Prometheus Fusion Perfection. Prometheus Fusion Perfection, 17 Aug. 2011. Web. 24 June 2013. .

31.           "Definition of Monoenergetic." - Wiktionary. The Wikipedia Foundation, 22 June 2013. Web. 24 June 2013. .

32.           Bussard, Robert W. "The Advent of Clean Nuclear Fusion: Superperformance Space Power and Propulsion." 57th International Astronautical Congress (2006). Web.

33.           Langmuir, Irving. "The Pressure Effect and Other Phenomena in Gaseous Discharges." Journal of the Franklin Institute 196.6 (1923): 751-62. Print.

34.           Merlino, Robert L. "Understanding Langmuir Probe Current-voltage Characteristics." American Journal of Physics 75.12 (2007): 1078. Print.

35.           "Systems Made to Measure Plasma Diagnostics for Research and Industry." Impedans. Impedians Inc., 2004. Web. 28 June 2013. .

36.           "Langmuir Probe." Wikipedia. Wikimedia Foundation, 16 May 2013. Web. 28 June 2013. .

37.           Chen, Francis F. Lecture Notes on Langmuir Probe Diagnostics. 2003. MS. Mini-Course on Plasma Diagnostics, Jeju, Korea.

38.           Nave, R. "Potential for Ring of Charge." Hyperphysics. Georgia State University, n.d. Web. 08 July 2013. .

39.           Liao, Sen-ben, and Peter Dourmashkin. "Example 2.4: Electric Field on the Axis of a Ring." Visualizing E&M. Massachusetts Institute Of Technology, 2004. Web. 8 July 2013. .

40.           "Obama's 2014 Science Budget: Research Gets Some Help, and Hurt." Science Insider. Science Magazine, 12 Apr. 2013. Web. 08 July 2013. .

41.           "Alcator C-Mod." Wikipedia. Wikimedia Foundation, 24 June 2013. Web. 08 July 2013. .

42.           Mott-Smith, H. M., and Irving Langmuir. "The Theory of Collectors in Gaseous Discharges." Physical Review 28.4 (1926): 727-63. Print.

43.           Cartwright, Jon. "Creating Monoenergetic Electron Beams on a Tabletop." Physicsworld.com. Physics World, 13 Dec. 2006. Web. 11 July 2013. .’

44.           Preliminary Results of Experimental Studies from Low Pressure Inertial Electrostatic Confinement Device

45.           "Defintion Of Monoenergetic." Answers.com. Answers, 2010. Web. 24 July 2013. .

46.           Carr, Matthew. "Low Beta Confinement in a Polywell Modeled with Conventional Point Cusp Theories." Physics of Plasmas 18.11 (2011): n. pag. Print.

47.           Alex Kline, Private communication. 18 July 2013

48.           Chen, Francis F. "Langmuir Probe Analysis for High Density Plasmas." Physics of Plasmas 8.6 (2001): 3029. Print.

Appendix: Modeling Force

1. Modeling the ring force. To model the force you need the magnetic field at the center of one ring.  The way to estimate this is shown below.

This shows a maximum field of 0.82 Teslas.  This is sensible, test four reached 0.16 Teslas.  Each ring is treated like an identical bar magnet.  This model ignores the side fields.  The force that would push apart two north poles is given by Gilberts’ model [22].  This is shown below with substitutions.

This model predicts 68 Newtons of force on one ring.  The actual amount is certainly higher given the presence of the other four magnets.  But this only occurred when the device was at peak power and no experiments reached this high.

Test I: Beam

1. How do you model a ring in the Polywell?  A ring is a donut shape.  This is a torus and it is too difficult to model.  Treating it like a disc is far simpler.  A simple equation can be used to model the electric field from one disc.  This equation applies only along the x-axis.  This is shown below [38, 39].  This equation works great - but you must have the charge on one disc.

 2. How do you estimate the charge on one disc?  Test I, measured a voltage of 125 in polywell center.  This voltage was made by six discs of charge, each two inches away. So by reorganizing the equation above, we can estimate the charge on one disc.  It is a 1.78E-10 positive charge.  This calculation is shown below.

3. What is the field made by two discs?  This is solved using superposition.  The field for each ring is plotted.  Then they are added together.  The field swings between 850 and 560 volts per meter and it goes negative to positive.  The electrons start at the filament and move into the center.  At the center they touch the probe.  The field was mapped out in excel and plotted below.

4.  What is the drift velocity at the probe?  The electrons start with no velocity.  They feel a Lorentz force which draws them towards the rings.  They accelerated towards the rings building up speed.  The electron can then be modeled using Newtons laws of motion.  This was done using excel.  The electron speed was found every two millimeters.  These equations are shown below.  The model says the electrons are moving about 1.7E6 meters per second when they get to the rings.  Khachans group measured about three times this in experiments.  This is a pretty nice agreement.

5. What is the velocity distribution used in test I?  Langmuir worked out this expression.  The electrons are moving along the x-axis in a beam.  The expression has four variables.  First is the radial velocity, which is directed along the axis.  Second is the tangent velocity, which is perpendicular to the axis.  The drift velocity is third - which is the overall beam speed.  Finally, there is theta.  This is an angle made by the probe.  A figure shows this better than any explanation. 

Using this geometry, Langmuir worked out this velocity distribution for a general beam [42].  The team had to tweak this equation to account for their probe.  The probe made an angle with the beam – theta – and this had to be worked into the math. 

6.  How is this combined with the probe expression?  This equation must be combined with Langmuir’s’ expression for wire probes.  The combined math predicts the IV curve.  This is a pain and it must be streamlined.  We substitute the values from the paper to clear up all the constants.  The plasma potential was 125 volts, the electron temperature was 9,279 Kelvin, the electron speed was 6.05E6 meters per second and the electrons density was 1E14 electron per square meter.  In addition 4.5 milliamps of electric current was shot at the rings.  With these constants added in, a manageable double integration remains.  This math is shown below.

We and Dr. Khachan could not find an analytical solution to this expression.  The equation needs to be solved numerically.  The numbers shown here are for SI units.  We attempted to do this using excel and MATLAB - but stopped due to time constraints.  Some of Khachans data can be download here.  All the IV curves were smoothed with a Savitzky-Golay filter. Test I data is shown below.

Test II: On or Off

1. What is the expression for velocity inside the cloud?  When turned on, the polywell creates an environment in the center.  In the center, there is a magnetic null point.  The electrons have a tight energy distribution.  They are moving in straight lines and are uniformly spaced.  These assumptions lead to an expression for electron energy.

The math is oriented around the wire.  The wire extends in the Z direction, and this is why the equation is integrated in that direction - to include all current to the wire.

2. Where does this distribution come from?  The team did simulations of electron motion.  This simulation was in a plane.  Electrons were emitted by four sources along each side of the device.  The simulated polywell had 7950 Ampturns in each ring.  The rings were biased 130 volts positive, which attracted the electrons to them.  The simulations revealed a spot in the center where 35 negative volts was measured.  Inside this simulation a monitor was inserted along one line.  The electron velocity was measured here.  Electrons moved in both the X and Y directions, but the monitor only measured the X velocity.  The field that was measured, along with the probe locations are shown below.

3. What does this distribution look like?  This monitor only measuring the x velocity.  This distribution is shown below.  The distribution has a sharply pointed peak around an average velocity.  What is important to note is, as the monitor moves outward, the average velocity rises.  This is surprising.  It an interesting result that Khachan does not discuss.  It supports the idea that the electrons are colder in the center.  The distribution slopes outward around this average, but it is uneven with more electrons moving slowly, rather than fast.  The “cloud velocity distribution” can be fitted to this velocity distribution.

4. How is this combined with the probe equation?  I am not sure I did this correctly.  To get a model which fit this data we need to combine the probe and velocity equations.  These are equations 11 and 3.  Unfortunately, the paper does not give us the solution.  They leave it to the reader to sort this out.  I have attempted to merge the two mathematically, but this may be flawed.  Feedback is appreciated.  Hopefully when Dr. Matthew Carr’s thesis is published, we can check this math.  These equations are shown below.

To combine these, it appears we need to bridge two coordinate systems.  One is the radial and tangent system for the probe.  The other is the X, Y and Z directions used to map motion inside the cloud.  We start by assuming that the Z and tangent directions point the same way.  They both point up the wire.  In integrating in Z, we account for all electron current as we move up the wire.  The wire is supposed to be an infinite cylinder, so we integrate to infinity in both directions.  Next, we assume that the cloud distribution can be put inside the probe expression - so long as it is in terms of radial velocity.  For this, we need to add an angle.  Using this angle one can write X and Y in terms of radial velocity.  These substitutions and assumptions are shown below.

This angle needs to be integrated around.  This integration will account for electrons hitting the wire from all sides.  To do a full revolution around the wire, we need to reach two pi radians.  The final expression becomes a triple integration.  We integrate around the wire, up and down the wire, and over the set of velocities which could reach the wire.  The equation is shown below.  The velocity integration is bounded by minimum velocity and infinity.  In practice, infinity should be the speed of light.  Any feedback on this is welcomed – this may be wrong.

This expression was fitted to data shown in the paper.  This is plotted below for when the polywell is on and when it is off.  The community is invited to see if this expression can be matched to this data.  This data can be download here.

Test III: Moving Probes

1.What probe data was made during this test?  There was spotty data given.  Four probe curves were listed in test III.  This is not a complete set and we do not know if these were the curves used in the final results.  This data is shown below.  This covers the full probe motion and can be download here.

1. What are typical numbers for these runs?  The paper gives one probe curve at 1.8 centimeters from the center and analyzes it.  This is done to illustrate a how the fitting becomes crummy as the probe moves into strong magnetic fields.  The results are shown below.  However, this is not relevant to the other measurements made in test III. This is because the local voltage is not the same as that given in the final plot.

Test IV:

1. How do you convert from B Field to Amp Turns?  The data in this plot is listed in terms of the magnetic strength.  This tops out at 26 militeslas.  The paper states the maximum field is located at the center of one of the rings.  This is wrong.  The strongest field is at the joint, where the rings come nearest to one another.  The conversion from magnetic field to amp turns is easy to do on the axis.  This is shown below using the Biot-Savart law.