Modeling Some Real Results

     This technology could change the world, if it works. It is a source of cheap, green energy. In fact, if Human civilization does not have a breakthrough technology like this; it is hard to see our civilization surviving. We are set to run out of all fossil fuels, and destroy our planets' ecosystem with climate change. If this works, it halts climate change and extends our energy resources indefinitely. This idea is screaming to be researched, reviewed and scrutinized. It is frustrating. For Polywellers, it is so hard to watch the current state of the world, when we know there is so many things that can be changed with a tool like this. A technology we know so little about; but that has so much potential. That is, if it works.

    Joe Khachan does not trust claims. He trusts data. It was the data that he measured which lead to the first Polywell science paper published in several years. The paper, which came out in the Physics of Plasmas Journal, in May 2010, is exciting. The polywell community now has access to some real findings about the machine. Regardless of the works' flaws or strengths. Regardless of wither the data is damning or encouraging – it is just exciting to see a polywell paper published in a science journal. This post is going to review that paper.

      We estimated that Dr. Khachans’ work cost a whopping grand total of 12 thousand dollars. Wow. We live in a world which just spent 75 million dollars on a musical about Spiderman; while spending 12 thousand dollars on research that could halt global warming [11]. Unbelievable. Regardless of cost, Joe will tell you that: ‘an ounce of experiment is still worth a ton of theory’ [2] He is right. Many great technologies, from the telephone to the automobile, started with simple setups, cheap materials and crummy performance. That is not important. What is important is; the principals being explored, the depth of analysis used and the innovation being tried. So if this setup is not great; it does not matter. We can still get useful information from this work.

     Dr. Khachans’ work fits with Dr. Bussards' work of the late 90’s and Mr. Suppes’ work of today. Indeed, these machines have similar designs and are of similar size.

Figure 1A) A picture of WB-3 developed by Dr. Bussards’ team from 1998 to 2001. This device had a radius of 10 cm. 5B) a picture of Dr. Khachans’ device developed at the University of Sydney, Australia, in 2009. This device had a width of 6 cm. 5C) a picture of Mark Suppes device built in Brooklyn New York, in 2011.

These similarities are encouraging. It reminds us that the Polywell is not a breakthrough. It is not a miracle discovery. This is the next step in natural progression of US funded research going back 60 years. People are excited because this technology seemed to come from nowhere. That’s not true. The current research is a logical step in a program which stretches back decades – and it has many more steps to go on its' path to a commercial reactor. It may yet still be a bad idea. What would help it along? If the majority of America accepted that this form of nuclear power was viable. If tomorrow 300 million Americans woke up and believed they could see commercial fusion energy in their lifetimes – that would be huge for everyone working on these projects. Please enjoy this review.

=================================================

Paper: “The dependence of the virtual cathode in a polywell on the coil current and background gas pressure.”

Published: The Physics of Plasmas Journal - May 2010

Summary of Joe’s Machine:

      The experimental setup consisted of a small polywell built out of machined Teflon. The rings were the size of a coffee cup. This is about 130 times smaller the Bussards' last machine [6]. The six Teflon rings were screwed together. Teflon is not ideal for a commercial reactor; but it will work well for this experiment. Inside the rings, ten turns of copper wire form the magnets. The rings were held inside a bell jar by an aluminum bar.

Figure 2: This is a picture of the Teflon rings used inside the Khachan group’s work. On the left the device is shown against a coffee mug for scale. On the right the device is shown off axis to show the screws and L joints which hold it together.


     The bell jar was glass container a foot and a half in diameter and several feet tall. One reason they could not test a larger machine was, because they did not have a larger vacuum chamber. The vacuum chamber pumped down to a pressure of 0.015 torr; compare that to 4E-7 torr in Dr. Bussards' last device [6]. They could not pump down to a lower pressure, because their cathode would not work at a lower pressure. The rings were wired to a 450 volt 2,500 amp power supply. By comparison, Dr. Bussards’ last device reached a voltage of 1,200 with 2,000 amps [20]. This power source was a bank of capacitors which could dump this electricity on the machine for burst, each hundredths of a second long. The capacitors were in series with a rectifier which ensures that direct current got pumped into the rings [7]. Lastly, there was a cathode tube which supplied a beam of electrons. The paper points out that this cathode is – in some ways - an improvement over Bussards’. In fact, they had published an earlier paper focusing entirely on this cathode device [8]. A diagram and picture of the machine they used is included in figures three and four below. This diagram was adopted from presentations, pictures and two papers. It is by no means complete or to scale.

Figure 3: A diagram of the experimental setup.  The polywell tested, was composed of six machined Teflon rings 6 cm in diameter.  Each ring had 10 turns of copper wire and was held together by L-joints and screws. 

Figure 4: A. this is a picture of the power source used in this work. Ten capacitors are attached in series with a silicon rectifier. The rectifier converts the AC current coming off the capacitors into direct current which is supplied to the Polywell. B. this is a picture of experimental setup inside the vacuum chamber used. There appears to be a cage around the outside of the chamber. I assume that this is the anode for the electron beam. The cathode may use thermionic emission. This is where a thin wire is heated by an electrical current. The heat knocks electrons off the surface of the wire [23]. The electrons travel as a stream from the cathode to the cage outside. As it does it passes right through the center of the polywell.

Materials Used in Setup:

     Teflon is an interesting choice for the rings. When choosing materials to make the rings, there are a number of issues to consider. Cost, machining, durability and outgassing are some examples. The two biggest problems for this work were electrical arching and disrupting the magnetic fields. There are two measures which can tell us if a material will create those problems: electrical conductivity and magnetic permeability. Pure Teflon has an extremely low electrical conductivity. This will help against arching. Pure Teflon also has a magnetic permeability which is 0.999:1 against vacuum [17]. That means from the magnetic field’s perspective: the Teflon is barely there. The material is also cheap and easily machinable. This is probably why Mark Suppes also chose it, for his device.

    There were other materials used here which concerned me. Bussard estimated that the electrons in his device recirculated about 100,000 times. Both Dr. Rider and Dr. Bussard stated that efficient electron recirculation would be essential [6, 12]. But, how can recirculation happen efficiently in this machine with so much metal surrounding it? This device has a Langmuir probe in the center, with an aluminum bar and metal wire hanging next to the rings. The rings themselves have metal screws and metal L-joints inside them. Allot of metal. This metal must disrupt the magnetic fields. The electrons ride these fields like cars on the highway. Disruption means bad recirculation and that should hurt the Polywell. Even if all the metal in the bell jar was held at nearly the same voltage – the materials should still have some effect. Table one below has the electrical conductivity and magnetic permeability for the materials surrounding the polywell.

Table 1: This is the electrical conductivity and magnetic permeability of materials used near the rings of the Polywell.

    Dr. Khachan needed strong, practical materials, which did as little as possible to disrupt the magnetic fields around the machine. At full power, each of the six rings has a field strength of 0.04 Tesla. The rings are placed six centimeters apart from one another. That means the rings are pushing each other apart with two tenths of a Newton force (see appendix). You need screws and L-brackets to hold this thing together. The device must also be held in line with the cathode and the aluminum bar works for this. The aluminum has a magnetic permeability close to vacuum; so from the magnetic fields point of view, the bar is barely there. Aluminum also conducts electricity – but if the bar was held at a uniform voltage, the electrons should have no reason to leak through the bar. The L-joints and screws have similar properties.

Improvements to this Design:

 

     I am sure Dr. Khachans’ group designed this Polywell around what was practical, buildable and affordable. However to maximize electron recirculation, there are at least a few improvements needed for a power reactor. First, the rings should probably look more like hula-hoops, rather than hub caps. This increases recirculation and minimizes all the metal the magnetic fields hit. This was part of Bussards’ revelation in the summer of 2005 – that electron recirculation was key – and this idea lead to WB-6 [18]. Another important change could be the ring spacing along the ring edges. Dr. Khachans’ machine has roughly 2.1 cm between each wire coil. A comparison between both devices is shown in cross section below.

Bussard stated that this was an important parameter. Bussard mentioned that this distance was some multiple of the gyroradi of the particles – and that the rings should not touch at the corners [19, 18]. This claim needs verification. Someone needs to figure out if 2.1 cm is a good distance or if it should be changed.

     Another change is the material used, to make the rings. WB-6 had a smooth metal shell. The lack of edges kept a charge from building up somewhere, leading to arching. The smooth surface also worked well with the swirling magnetic fields. Electrons could ride the B fields and not hit a metal edge on the rings. It is unclear what material would be best suited for the rings. People have mentioned cooled ceramic superconductors as well as tough tungsten carbide to withstand the neutron blowback. Teflon may have a place in the reactor chamber – given its electromagnetic properties. However, in practice Teflon can build up a charge in the chamber as well as brown when exposed to plasma. It is also contains gas pockets which can be hard to vacuum out [5]. This topic is open to discussion.

Can the Polywell Contain Electrons?

    Imagine a bucket. Now take a hose and spray water into the bucket. If your bucket has a hole, water will leak out. Dr. Khachan is testing wither the magnetic confinement can hold electrons. The Polywell acts like the “bucket”, the cathode serves as the “hose” and the electrons are the “water”. The cathode sprays a stream of electrons into the center of the Polywell, and Dr. Khachan is testing to see if they will be contained in the center. If contained, these electrons will form a virtual cathode. This paper tests how the virtual cathode changes with chamber pressure, current through the rings and injection energy. Given all we know about magnetic confinement of plasma, we would expect the fields to hold in the electrons. The first question is: how fast are you shooting these electrons into the machine? Water can emerge from a hose in a dribble or a jet – electrons can be injected at low and high energies. One of the ranges of energies used in this work, was a 10 milliamp beam from roughly 7.4 to 15 kilo electron volts.

The Electrons’ Worldview During One Test:

      Let us look at one test from the point of view of the electron. This is a summary of the electrons worldview- for all the detailed modeling which leads to this section, please see the appendix below. We choose to model one test condition from the paper. This condition was: 625 amps through the rings, 15 mTorr background pressure and a 15 KeV electron beam.

     For this run, Joe pumps his vacuum chamber down to a 15 mTorr pressure and switches on the device. Assuming the cathode uses thermionic emission, a wire is heated and an electron migrates to the wires’ surface and leaves [23]. From the moment it is emitted, the electron feels a Lorenz force. This force directs the beam towards the polywell rings. It does this, by creating an electrostatic field drawing the e-beam towards the cage at the edge of the vacuum chamber. The electron leaves the cathode. It moves slowly at first, but picks up speed as it “falls” down a 15 KeV voltage drop. The electron crosses the distance to the edge of the Polywells’ rings. From the electron’s point of view this is an infinite distance – relative in scale to a person traveling to Pluto seven times! The electron should cross the distance without hitting any background gas, because the mean free path of the gas is about six meters. The electron arrives at the rings’ edge in roughly 7.2 nanoseconds. At this point the average electron should be flying at a speed of around 2.5E7 meters per second. By this time, 625 amps are pumping through the polywell’s rings creating about a 0.078 tesla field, where each ring generating one sixth of that. The electron starts to “feel” that magnetic field – and it experiences a, Lorentz force with the added magnetic component. This added force is directed perpendicular to both the net velocity and the net magnetic field.

     The paper states that the cathode emits a single collimated, monoenergetic electron beam. The beam is 10 milliamps. In this case the Polywell should capture the electrons. The beam was also modeled – using some specifications from a previous paper – as having a bell curve of energies. In this case as well, the Polywell should capture most of the electrons. Oddly, it is the slower moving electrons in the beam which fly right through the device and are lost. This is because the magnetic force depends both on the electrons velocity and the machines magnetic field. The slower the velocity, the lower the magnetic Lorenz force the electron feels pulling towards the polywell. Too slow, and the electron flies right through. However, for most of the electrons, the magnetic force at the rings’ edges is stronger than the electric force driving the beam. Hence, these electrons get caught. I have gone through the detailed mathematics to show all this, in the appendix.

     The electrons start filling up the Polywell. Modeling fill up is beyond the scope of this post, but some description about how this mechanism is thought to work is provided. It takes some time for the electrons to fill up the center. The data from the paper indicates that this fill up time - depends on the drive current. The paper theorizes that there a “resonance current” for this device. They call it a threshold value. This means the device catches the most electrons at a specific magnetic field strength. If your starting current is too high, it takes time for the current to decay. As the current decays, the magnetic field strength lowers until the “resonance current” is hit. At that point the number of electrons in the center reaches a maximum. At the peak, there were about 10 billion electrons contained in the center during the 625 amp run. If the average lifetime of an electron is 100 microseconds in this machine, the average electron should make 42,000 trips around the rings. By contrast Dr. Bussard estimated that his electrons recirculated about 150,000 times. There are plenty of reasons to expect that Dr. Khachans’ machine would have a lower recirculation rate. This mechanism is just an idea. This being an early Polywell paper – there is fair reason to think much more will be discovered. In the end, the device may or may not have such a “resonance current”.

Magnetic Fields’ Effect on the Electron Cloud:

     Let’s start with examining some data from the paper. The graph below includes typical data from two runs. The run we modeled above, is also drawn in, as it is expected to have looked. The time to peak, is highly questionable - but we included it for the reader's sake. For a model of how this was calculated, please see the appendix. For each run, there are three numbers. These are: the chamber pressure, the injection energy and the drive current. Unfortunately the paper does not make clear all three numbers for all three runs. We do know that, the pressure varied from 15 to 35 mTorr.

The graphs really show this “resonance current” condition. When 115 amps is applied, it creates a magnetic field which is too strong. A little time passes, the current dissipates and it hits the specific magnetic field for this device. At this point, the amount of electrons caught peaks; and the voltage dips sharply. If this mechanism is true, then it makes sense that the 625 amp run would take so much longer. It takes more time for the current to dissipate. It should be noted the time to the 625 amp peak was not given – it was estimated using other data. There is strong indication this value is incorrect, but it is drawn in to show to the reader the “resonance current”. Please see the appendix for details.

   There is some indication that this mechanism is not a complete description of the data. The paper reflects this in the wording it uses: “…When the peak magnetic field (and the equivalent coil current) is above this threshold, potential well formation is generally not observed until after the field has decayed down to the threshold value, producing an…” (underline added). If the study found any data that contradicted this hypothetical mechanism, they were careful not to include it in this section. This is not uncommon in science - especially in the early papers on a topic. Researchers will limit themselves to a region of data, where clear patterns emerge. They will publish with that data, and leave the other regions to other groups. Since they do not know all the factor in play - this is a reasonable and accepted strategy.

The Supposed Physical Mechanism:

    If this mechanism is correct, then the physics driving it can be explained using magnetic mirror theory. Charged particles bounce off magnetic mirrors. Magnetic mirrors are the main method researchers use to contain plasma in fusion reactors. To establish a magnetic mirror the electron speed must be kept well below the variations in the magnetic field. This is kind of like: keeping the energy of the electron much lower than the containing energy. In this state, a number of equations are true. First, there is an inequality which holds. This inequality is shown below along with a diagram explaining different operating conditions. The image on the left is where a dense field contains the electrons. The image on the right, is a light field, where electrons can escape. You can tell the difference if you compare the electron velocity against the changes in the magnetic field - that is the very important inequality. You will notice that one of the values - the gyro frequency of an electron – is shown and a constant. It can be easily found online [24]. The gyro frequency is how fast an electron spins around a magnetic field.

    This may explain the “resonance current”. At high currents, like 625 amps, the fields are really dense. This means the characteristic length is really small and the above inequality fails. The magnetic mirror is terrible and electrons are poorly trapped. This is why the voltage is so low at the beginning of the high current data. Then the fields dissipate. As they dissipate the fields become less dense. The characteristic length gets bigger, and the inequality starts to hold in places. This means the magnetic mirror gets stronger and electrons are trapped better. This physical process peaks at the “resonance current”. At this point the magnetic mirror is really strong, many electrons are caught and, the voltage dips sharply. This is the papers claim anyways. Magnetic mirrors are well studied, and have been for the past several decades. If Dr. Khachan can link device behavior to this physical phenomenon then a number of equations, assumptions and behaviors can be ascribed. It is actually a very complex topic and if I had made a misstep in explaining it, please let me know. It is important to understand and connect all the parts of it. The important message here is: the paper has advanced a physical process which explains what they observed.

    The paper theorizes that: not only is the magnetic mirror changing in time, but it is also changing in physical space. At any given moment in time there are regions within the device where the inequality holds and were it fails. These regions are roughly sketched in an example field below. The mirror fails along magnetic cusp lines. Otherwise, the regions are arbitrarily drawn.

This is a more refined picture then the magnetic mirror line included in the post: “The debate over electron behavior”.  In that post, the cusps were not included.  In the regions described above the electron is moving slow compared to changes in the magnetic field.  The electron velocity is low, against changes in the magnetic field.  This sets up a special region of the magnetic field.  In this region the magnetic moment is constant.  The moment is a measure of how the electron reacts to the magnetic field.  The equation for the moment is shown below.

When these conditions are met – constant magnetic moment and the inequality – one can treat regions of the Polywell like magnetic mirrors.

A More In-depth Description of Magnetic Mirrors:

   I found a very good description of magnetic mirrors in “Introduction to plasma physics” on page 29, by FF Chen [27]. I combined it with Dr. Fitzpatrick’s’ notes on magnetic mirrors [26]. Imagine an electron moving from a weak magnetic field to a dense magnetic field. This is shown below.

The magnetic mirror region has three important properties.  The first is that the velocity of the electron is low compared to the changing magnetic field.  This is what the inequality above is telling us.  The second is that the magnetic moment remains constant.  The third assumption, is that the total energy remains constant.  The electron is rotating around the magnetic field lines.  This is due to the magnetic component of the Lorenz force, which points inward.  The electron takes a corkscrewing path - where the radius gets tighter as it moves deeper.  The electron is moving into a higher density field, with a higher energy density.  This is sort of like pushing a rock uphill.  In that case, the rock is transferring its kinetic energy to potential energy.  The electron is doing much the same in this example.  The potential energy is increasing and the magnetic moment is constant.  The only way for this to happen is if the velocity perpendicular to the field increases as well.  You can work this out mathematically.  The equations involved are shown below.

    By why does this cause the electron to reflect? As the electron moves deeper into the field, the potential energy and half the kinetic energy are both on the rise. The only way for the total energy of the system to remain constant is if the kinetic energy parallel to the field declines. The velocity parallel to the field must decline. This is the key to the mirror idea. Eventually the velocity parallel to the field drops to zero – and the electron turns around. Where this happens inside the device constitutes the magnetic mirror line. This physical process is the key to magnetic mirrors, such as the MFTF and the TMX machines. For a more detailed analysis, including a longer list of the equations involved, please see the appendix.

Loss Cones and Mirror Ratios:

   Physicists are funny. They create fictitious entities to help them understand processes. This is true in the case of a loss cone. A loss cone is a way to display to someone what electrons would be lost in a magnetic mirror. A picture of a loss cone is shown below [27]. Oddly, it is the slower moving electrons that are lost. These electrons have velocities which would fall inside the cone. The slower moving electrons do not experience as strong a magnetic force inside the Polywell. Hence, they are lost. There is a simple equation which determines the angle of the loss cone. Unfortunately, the papers' equation and FF Chen's equations differ, so both are listed. If someone could explain the difference, I would really appreciate it.

     One of the most critical parameters when examining a magnetic confinement device is the mirror ratio. The mirror ratio is an important measure of how well a device confines its plasma. Rider used a low mirror ratio in his general critique paper [29]. The paper he referenced was “Particle loss rates from electrostatic wells of arbitrary mirror ratios” published in 1984 by Peter Catto. That is a good paper to read when looking at mirror ratios. What Peter was after was a mathematical way to connect a mirror ratio to the loss rate of electrons. One of the bits of information I was hoping to come from Dr. Khachans work was what mirror ratio he measured. Then we can compare what value Rider used to what value Khachan measured. If the Whiffle ball effect is happening, we would expect the ratio to be very high. Unfortunately, this work does not give a ratio. FF Chen provided one definition of the mirror ratio. However since the loss cone definition is slightly different than the paper, it is unclear which equation is correct. I did not have time to clear this up, if someone could assist here, I would appreciate it.

Background Pressure and Injection Effects:

     Potential well formation was measured as a function of three background pressures. The ring current, for all runs, was kept at 625 amps. The well disappeared at 35 millitorr, suggesting that pressure was not low enough. The data, showing the measured potential well for different pressures and injection energies is below [28].

The most important conclusion from this section is that the data suggests that lowering the pressure improves the potential well, one can create. The other conclusion advanced is that the well improves, with more powerful injection energy. The data suggests both these statements. However, it seems too early to substantiate either statement, on so few test conditions.

Conclusion:

     If you have read over this post then you understand how complex this reactor is. It is complex to explain, to model, to build and to observe. It is complex for the experts as well. We are sure there are mistakes somewhere in this post. If you find some, please let us know. It was difficult to work through this material and we are not perfect. Give us feedback – but don’t degenerate into name calling. Sign up for the blog.

    The world is rapidly running out of fossil fuels. Global warming from CO2 emissions is changing the biosphere. The population is on track to break 7 billion people. Everyone is looking for cheap, clean, abundant energy. There are a host of promising technologies out there that might deliver this. If it works, the Polywell should be on this list. We need to know if it works. We need to fund research. We need much more data. Ultimately, this may turn out to be a bad idea, but at least we will know for sure.

Appendix I – Modeling the 15 KeV, 625 Amp, 15 mTorr, Test

1. Estimating the mean free path. I assume the gas particles have a Boltzmann distribution. We know from the work that at pressure of 20 mTorr the mean free path is ~5 meters. From this we can estimate the gas particle diameter and the mean free path at 15 mTorr, which is about 6.6 meters.

2. Modeling the electron flight.  Here are the equations I used to model the flight of the electron.  First I used a simple proportionality to find what the distance from the cathode to the rings is, relative to the size of the electron.

This proportionality says that to the electron, crossing this distance is like to a person traveling seven times to Pluto. It takes roughly 7.2 nanoseconds to reach the rings (see below).

3. Modeling the Magnetic fields. The modeling for the magnetic field came from the Biot-Savart approximation. I modeled the electron coming in on a straight line through the center of the rings. I used a very simple equation which assumes a constant current, ignored the effects of the other rings or the other electrons [21]. This is a very simple approximation.

4. Modeling the Magnetic and electric field for a monoenergetic, 10 milliamp beam. Modeling the electrostatic field was somewhat trickier. The paper states that the beam was a 10 milliamp, collimated, monoenergetic beam. I also modeled the beam as a Boltzmann distributed beam, see below. I estimated the beam length at 30 cm from the image provided and previous papers describing the cathode [16]. This is shown schematically below.

The paper states the electron injection energy was 15 KeV.  This means one electron fell down a 15 kilovolt drop to pick up this energy.  This is where things get somewhat murky.  Was the field 15 kilovolts per meter?  If so, this means the electron saw 4500 volts between the cathode and the cage.  Was the field 50 kilovolts per meter?  If so, the electron saw 15,000 volts between the cathode and the cage.  Additionally, from an earlier paper, Dr. Khachan states that the energies the ions experience in his beams are ~30% that of the applied cathode potential [23].  This turns out to be a very important issue.  The different possibilities and what they mean are worked out below.  In each case, I modeled electron flight using both the electric and magnetic fields it would experience.  I assumed a 1 degree difference between the magnetic field vector and the velocity vector.  If someone knows what the actual field applied between the cathode and the cage was, please let me know.  I compared the magnetic and electric Lorenz forces at the rings edge - and used this to determine if the electron would be "caught" or not.

As one can see from above, in all cases the magnetic pulling force of the Polywell is stronger than the electrostatic force driving the beam. Therefore in each case the polywell catches the electrons. I take the applied voltage as 15kv per meter. I assume the cathode uses thermionic emission to generate the electrons [23]. If the beam is at 10 milliamps then it is generating 6.2E16 electrons per second. Each electron starts it flight with no kinetic energy. The beam itself is about a centimeter in diameter [16] and it has a rough electron density of 1E8 electrons/cm^3 [13].

5. Modeling both the electric and magnetic fields. I can explain how I got the above chart. The magnetic field calculated above was for an electron flying in on axis. In this situation, the electron should feel no magnetic force. This is because the velocity vector and the magnetic field, line up. The rings are set up to be six south poles pointed inward. Hence, the electron sees magnetic field lines pointed inward. To correct for this problem, I modeled the electrons just off axis. I assumed a three degree difference in the direction of the velocity and magnetic vectors. This directional difference allows the electron to experience the magnetic field. The flight of the electron is controlled by a Lorenz force. This force has a magnetic part emanating from the rings and an electrostatic part, pulling the beam towards the cage wall. The electric field I ended up using was 15 kilovolts per meter. The equations and results are shown below.

     These equations were applied iteratively. The electron was modeled each time it moved 1 centimeter closer. This model predicts the electron will reach a speed of about 2.5E 7 meters per second and will reach the rings in 7.2 nanoseconds. Incidentally the magnetic part of the Lorentz force points a little off axis from the direction of the beam. This means that the electron will take a slightly curved path as it travels towards the rings. It also should be noted, that this model is for the device at startup, and the other five electromagnets are ignored. After startup, the electron should feel the aggregate magnetic and electric fields of the electrons swirling inside the device and each of six 0.013 Tesla electromagnets.

6. Modeling the electron Beam as a bell curve. It is hard to believe that the beam energy does not spread out as it crosses the space. The paper states that the cathode produces a collimated, monoenergetic beam. There was no time to analyze the reference paper to get the details of this beam (ref 8). This does not matter, either way. If the electrons can be treated as all having the same energy - then they still should be captured by the device. As will be shown below, if the electron spread out, they should be captured as well.

     As the beam crosses to the Polywell, the electrons bump into one another. The magnetic part of the Lorenz force is pointing off axis creating a slightly curved path for the electrons to traverse. Hence, they should run into one another. As a consequence their velocities should spread out. When the beam reaches the rings, the electrons should have a bell curve of velocities. This is explained in the diagram below.

There is a way to mathematically estimate this spreading. The speed, time, and energy calculated above will be used as the “average” electron in that beam. The rest of the beam can be modeled as a bell curve around these energies and speeds. We will try to define that bell curve.

     The first step in modeling this spreading is to define the fundamentals of this process: the typical time for electron bumping, the number of electrons and the typical energy exchanged. I am going to assume that each bump transfers 3.2E-21 joules of energy, about the energy the average particle has at room temperature. This assumption is debatable; but the electrons are contained at room temperature. Each transfer of energy will form a basic energy level for our system. I am also going to assume that each electron bump takes 320 attoseconds [15] the same amount of time it takes an electron to jump from atom to atom. People can argue with me on these assumptions. The model predicts that it should take the electrons 7.2 nanoseconds to reach the rings. This translates to 2.3E7 of time steps in this system. After 23 million interactions across the beam it is hard to assume the beam is monoenergetic. An earlier paper listed this beam as a centimeter in diameter [16] and as having a rough electron density of 1E8 electrons/cm^3 [13]. If we look at a half centimeter long chuck of the electron beam as it reaches the rings, it contains 1.6E8 electrons.

     The simplest way, I found, to model how the velocities of electrons inside the beam change over time, was modeling it like a Wiener process. A Wiener process happens when at each time step a variable “spreads out more”. At each time step, the range of the variable gets larger – while variable’s distribution remains shaped like a bell curve [10]. There is a simple equation for this process, as well as the resulting bell curve of electron speeds is given below.

The graph above is slightly off, it shows the bell distribution around one average. In reality the bell curve should be spreading out and moving from left to right. That is because earlier in the beam the electrons have a lower average velocity. This predicts that the slowest electrons are traveling 1.4E7 meters per second and the fastest are traveling 3.18E7 meters per second. I applied both speeds to the Lorenz force equation, to see if the magnetic component was stronger than the electrostatic component (see below). At both the high and low speed, the electron should get caught by the device.

7. How Do I test for electrons being caught? This question could be rephrased: which component of the Lorenz force is stronger, the magnetic or electrostatic part? This can be answered by rearranging the Lorenz force equation and applying it to an electron as it reaches the rings. This equation is shown below. An example calculation is shown.

For this equation, I assumed that by the time the electrons reach the edge of the rings they feel all six electromagnets. I also assumed only a one degree difference between the velocity and the B fields. That is a low degree difference, for many electrons this would be higher. Oddly, it is the slower electrons which are not “caught” by the Polywell. This is because of the magnetic force depends on the electrons’ velocity and the machines’ magnetic field strength. Too slow of a velocity, and the magnetic force is too low to overcome the electric field driving the beam.

   This model quickly falls apart after startup. As the polywell fills, with electrons the field from ~10 billion electrons should affect the aggregate magnetic fields. Beyond these simple conclusions, a model of the electron’s flight becomes futile. I would love to work up a simple model in comsol or a correlating physics modeling software – but this becomes a question of how accurately a model do you need. We know from Dr. Bussard that, ultimately building the device is far easier then modeling it. For our purposes these simple equations suffice. Several people have made models of the device before – including FoxRoger, HappyJack and Indrek.

6. Figuring the number of trips around the rings. This calculation is easy given all the modeling that has been done above. If you figure the average transit for the electron is 6 centimeters, you know the average speed is 2.53E7 M/S and if the lifetime is 100 microseconds then the electron should make 42,000 transits around the ring. By contrast Dr. Bussard estimated that his electrons recirculated 150,000 times in WB-6.

7. Figuring the number of electrons contained. This equation and model have been used in past posts. The center of polywell is treated like a point charge and it is assumed that at the cathode there is no charge felt.

This works out to 1E+10 or ten billion electrons. As a reference point, it was estimated that WB-6 on one published run held 1E+13 electrons. Alternatively one could use gauss’s law to make a rough approximation of the number of electrons inside the device.

Appendix II – Modeling the Reactor:

1. Modeling the repulsive strength. Here is the equation I used to estimate the magnetomotive force on the rings, when under full power. The machine had a 0.25 Tesla field – this is the power of each ring added together. Divide by six, and each ring should have a 0.041 Tesla field. This is with 2,000 amps pumping through it. The equation used below predicts the field strength felt in the center of each ring, solely due to that ring.

This equation predicts a single rings’ field strength of 0.0418.  This is in agreement with what we expect.  I modeled each of the rings like circular bar magnets and I ignored the side fields.  Therefore, the calculation I did was two bar magnets with the above field strength placed six cm apart from one another.  This is obviously an estimate; but it should give us some feel for the forces felt inside the machine.  From Gilberts’ model [22]           

This equation works out that each ring experiences two tenths of a Newton, of force. The actual amount is certainly higher given the presence of the other four magnets not accounted for in this calculation.

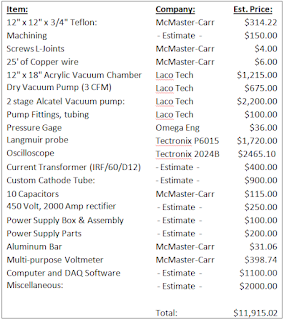
2. Figuring the Cost. Here is a rough cost estimate for Dr. Khachans’ work. This table may easily be lacking some equipment – and several values are estimates based on similar products and services.

3. Approximating the time to minimum.  We needed to estimate the time it took to reach the 625 amp peak.  This estimation could be flawed.  The reader needs to understand that.  There are two figures from the paper which we looked at.  The first is figure 5A.  It shows the potential well minimum as a function of the applied current.  The predicted voltage for 625 amps is shown, as well as the voltage actually measured.

In this case a prediction is clearly incorrect. It can be assumed the researchers saw that the 625 amp data did not follow a predictable pattern. Hence, they did not try to ascribe a pattern to all of the data. This is not uncommon in science: published papers generally try to limit the scope of analysis to data where they can make definitive statements. They leave the other data for someone else to deal with. In this case, the pattern is: a sharp step around 100 amps. This is their threshold value, or as we have been calling it the “resonance current”. This indicates that an interesting physical process is happening at that current. They believe this process is the magnetic mirror phenomenon, as described above. The 625 amp data does not necessarily follow this pattern – so they were careful about what statements they could make.

       Given that the above prediction is flawed, our estimate for the time to the 625 Amp peak must be taken with a grain of salt. The paper does not provide this value. It is estimated from figure 5b, which shows the time to the minimum for different starting coil currents. This is shown below.

From the equation drawn in, the time to minimum is estimated to be 100 milliseconds. This value was used to create the data drawn in the plot above.

4. Analyzing the magnetic mirror. This analysis combines a number of equations from Dr. Fitzpatrick’s’ notes, FF Chen and online research. Fortunately, all of the equations used turn out to be self-consistent. The idea was to analyze what happens when an electron moves from a low density field to a high density field. We are focusing on the region of the field where the magnetic mirror assumptions hold. This region has three interesting properties. First, the rate of electron motion is much smaller than the rate of magnetic field change – relative to the gyroradius. Second, the magnetic moment - the measure of how the electron will respond to the magnetic field – is constant. Lastly, the total energy of the electron is treated as constant. I found a number of equations for this system and wrote them all down. This was a complex system, with many variables and equations and I was worried the math would not work out. It did. Not every equation was included, however, including the centripetal force and the magnetic component of the Lorenz force, inside this hypothetical system. Looking over the math, the only way for the magnetic field to increase and those three conditions to hold was if the velocity perpendicular to the magnetic field declines. It is supposed to decline to zero. At this point it turns around. This represents the magnetic mirror line. The equations used are shown below, with a small explanation between each [25,26,27].

Works Cited:

1. Fusion Research Projects - Fusion Group - The University of Sydney." School of Physics – The University of Sydney. 10 June 2010. Web. 09 June 2011. .

2. Barry, Tony. "An Interview with Dr. Joe Khachan." Www.tonybarry.net. Tonybarry, 17 May 2008. Web. 10 June 2011. .

3. Strout, Joe. "Q&A with Joe Khachan about Copper Coil Polywell « Prometheus Fusion Perfection." Prometheus Fusion Perfection. Prometheus Fusion Perfection, 1 Apr. 2010. Web. 10 June 2011. .

4. "Dielectric Constant of Teflon." Teflon® AF Properties. Dupont, 2011. Web. 12 June 2011. .

5. Chris, and Thomas Ligon. "Advice on Teflon." Web log comment. Talk-Polywell. 21 Feb. 2011. Web. 10 June 2011. .

6. Bussard, Robert W. "The Advent of Clean Nuclear Fusion: Superpreformance Space Power and Propulsion." Proc. of 57th International Astronautical Congress, Spain, Valencia. IAA & IAF, 2006. 12. Print.

7. "Silicon-controlled Rectifier." Wikipedia, the Free Encyclopedia. Wikipedia Foundation, 29 May 2011. Web. 12 June 2011. .

8. Kipritidis, J., and J. Khachan. "Absolute Densities of Energetic Hydrogen Ion Species in an Abnormal Hallow Cathode Discharge." Physical Review (2008): 066405-1-66405-9. Print.

9. Formula, Euler's. "Permeability (electromagnetism)." Wikipedia, the Free Encyclopedia. Wikipedia Foundation, 9 June 2011. Web. 13 June 2011. .

10. "Wiener Process -- from Wolfram MathWorld." Wolfram MathWorld: The Web's Most Extensive Mathematics Resource. Wolfram Research Inc., 18 May 2011. Web. 06 July 2011. .

11. 'Spider-Man' Musical Opens: What Critics Said. Perf. George Pennacchio. KABC-TV Los Angeles, 2011. Online.

12. Rider, Todd H. Fundamental Limitations on Plasma Fusion Systems Not in Thermodynamic Equilibrium. Thesis. Massachusetts Institute of Technology, 1995. Print.

13. Khachan, J., and A. Samarian. "Dust Diagnostics on an Inertial Electrostatic Confinement Discharge." Physics Letters A 363.4 (2007): 297-301. Print.

14. "Alloy 304 - Austenitic Stainless Steel Plate - Sandmeyer Steel." Stainless Steel Plate and Nickel Alloy Plate Products By Sandmeyer Steel. Sandmeyer Steel Company. Web. 14 June 2011. .

15. "Attosecond." Wikipedia, the Free Encyclopedia. Wikipedia Foundation, 4 May 2011. Web. 06 July 2011. .

16. Kipritidis, J. "Applications of Doppler Spectroscopy in H2 to the Prediction of Experimental D(d,n)3He Reaction Rates in an Intertial Electrostatic Confinement Device." Physical Review 79.026403 (2009): 026403-1-26403-8. Web.

17. Keyser, Paul T. "Magnetic Susceptibility of Some Materials Used for Apparatus Construction (at 295 K)." Review of Scientific Instruments 60.8: 2711-714. 1989. Print.

18. Bussard, Robert. "Robert W Bussard: Electrostatic Confinement Fusion." Rex Research. 2007. Web. 23 June 2011. http://www.rexresearch.com/bussard/bussard.htm

19. Should Google Go Nuclear?" R.W. Bussard. Google Videos. 9 Nov. 2006. 20 Feb. 2009 .

20. "Should Google Go Nuclear?" by Mark Duncan, 12-24-2008, www.Askmar.com, page 13.

21. Nave, R. "Magnetic Field of a Current Loop." Hyperphysics. Georgia State University. Web. 26 June 2011. .

22. "Magnet." Wikipedia, the Free Encyclopedia. The Wikipedia Foundation, 22 June 2011. Web. 26 June 2011. .

23. "Thermionic Emission." Wikipedia, the Free Encyclopedia. Wikipedia Foundation, 2 June 2011. Web. 29 June 2011. .

24. "Plasma Parameters." Wikipedia, the Free Encyclopedia. The Wikipedia Foundation, 14 Feb. 2011. Web. 19 July 2011. .

25. "Gyroradius." Wikipedia, the Free Encyclopedia. The Wikipedia Foundation, 3 July 2011. Web. 19 July 2011. .

26. Fitzpatrick, Richard. "Magnetic Mirrors." Home Page for Richard Fitzpatrick. The University of Texas at Austin, 31 Mar. 2011. Web. 19 July 2011. .

27. F Chen, Francis F. (1984). Introduction to Plasma Physics and Controlled Fusion, Vol. 1: Plasma Physics, 2nd ed.. New York, NY USA: Plenum Press. ISBN 0-306-41332-9. Pages 29 - 30

28.Carr, Matthew, and Joe Khachan. "The Dependence of the Virtual Cathode in a Polywell™ on the Coil Current and Background Gas Pressure." Physics of Plasmas 17.5 (2010). American Institute of Physics, 24 May 2010. Web.

29. Rider, Todd H. "A General Critique of Inertial-electrostatic Confinement Fusion Systems."Physics of Plasmas (1995): 1853-872. Web.